Datasheet (uk) sg2000l a_b
SG 2000 L / Komp. A+B High quality, vey thin liquid fast casting resin system SG 2000 L Comp. A SG 2000 Comp. B Applications Properties • Vacuum forming tools• Mould probes for trimming of prototypes Processing data Hardener SG 2000 L / Komp. A+B SG 2000 L Comp. A SG 2000 Comp. B Mixing ratio Physical data Properties Inspect. requirem. Sales units
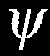 Fig 1: Equilibrium Growth, Employment, and Income Distribution
Appendix: Derivation of macroeconomic equilibrium
Fig 1: Equilibrium Growth, Employment, and Income Distribution
Appendix: Derivation of macroeconomic equilibrium